Area Between Two Curves: A Visual Guide

Understanding the area between two curves is a fundamental concept in calculus, offering insights into the relationship between functions and their graphical representations. Whether you're a student, educator, or professional, mastering this topic can enhance your problem-solving skills and deepen your appreciation for mathematical principles. In this guide, we'll explore the area between two curves through a visual and step-by-step approach, making it accessible for both informational and commercial-intent audiences. (calculus tutorials, area under curves, mathematical integration)

What is the Area Between Two Curves?

The area between two curves refers to the region bounded by the graphs of two functions, typically over a specified interval. This concept is crucial in calculus for calculating volumes, areas, and other applications in physics, engineering, and economics. By visualizing and computing this area, you can solve real-world problems efficiently. (calculus applications, bounded regions, function integration)

How to Calculate the Area Between Two Curves

To find the area between two curves, follow these steps:

• Identify the Functions: Determine the two functions, ( f(x) ) and ( g(x) ), whose curves you’re analyzing.
• Define the Interval: Establish the limits of integration, ( a ) and ( b ), where the curves intersect or are bounded.
• Set Up the Integral: Use the formula:
  [ \text{Area} = \int_{a}^{b} [f(x) - g(x)] \, dx ] Ensure ( f(x) \geq g(x) ) over the interval.
• Evaluate the Integral: Compute the definite integral to find the area.

📘 Note: If the curves intersect within the interval, split the integral into subintervals to ensure accuracy. (definite integrals, interval limits, curve intersection)

Visualizing the Area Between Two Curves

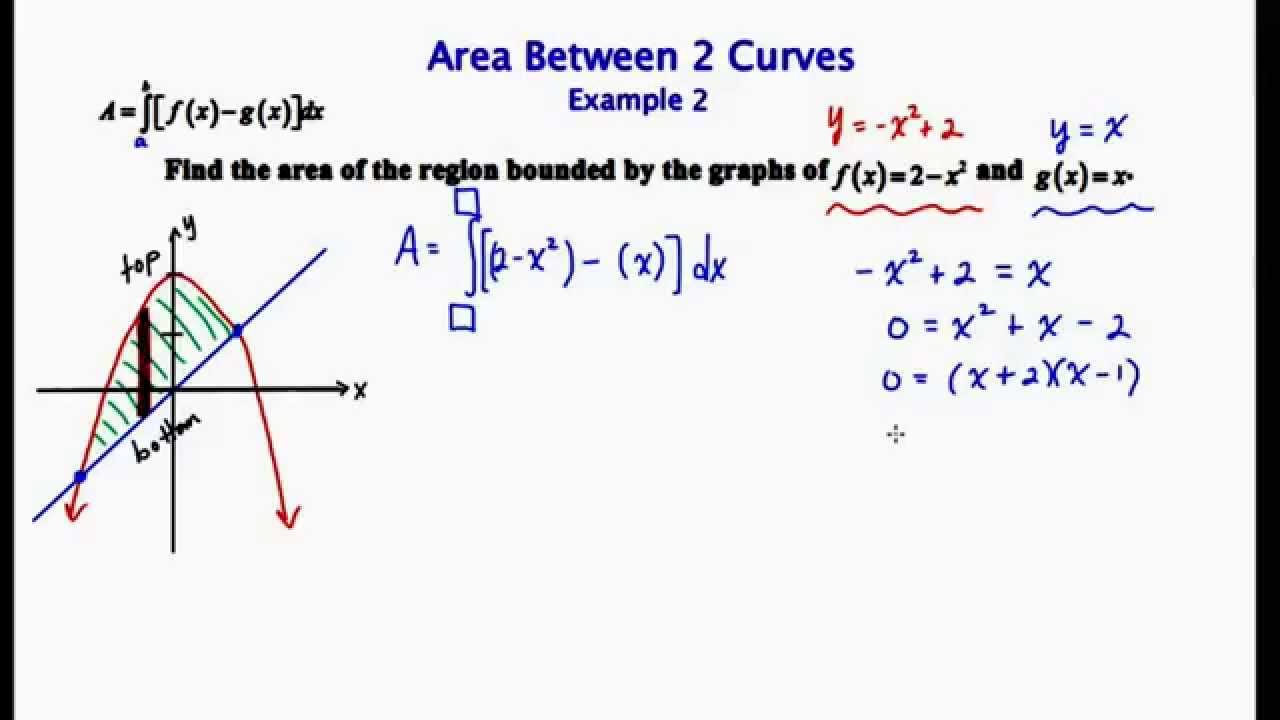
Visualization is key to understanding this concept. Graph the functions ( f(x) ) and ( g(x) ) and shade the region between them. Tools like graphing calculators or software (e.g., Desmos, GeoGebra) can help you see the bounded area clearly. This visual approach reinforces the relationship between the functions and the calculated area. (graphing tools, shaded regions, visual calculus)

Practical Applications of the Area Between Two Curves

This concept has real-world applications, including:

• Physics: Calculating work done by a variable force.
• Engineering: Determining material quantities in construction.
• Economics: Analyzing consumer surplus or producer surplus.

Mastering this skill opens doors to solving complex problems across disciplines. (real-world calculus, work calculation, economic analysis)

Checklist: Steps to Calculate the Area Between Two Curves

• Identify the functions ( f(x) ) and ( g(x) ).
• Determine the interval ( [a, b] ).
• Set up the integral ( \int_{a}^{b} [f(x) - g(x)] \, dx ).
• Evaluate the integral to find the area.
• Visualize the result using graphing tools.

The area between two curves is a powerful tool in calculus, bridging theoretical concepts with practical applications. By following the steps outlined in this guide and leveraging visual aids, you can confidently tackle problems in this area. Whether you're studying for exams or applying calculus in your profession, this knowledge will prove invaluable. (calculus mastery, problem-solving skills, mathematical visualization)