Understanding Hooke's Law: The Role of K Constant
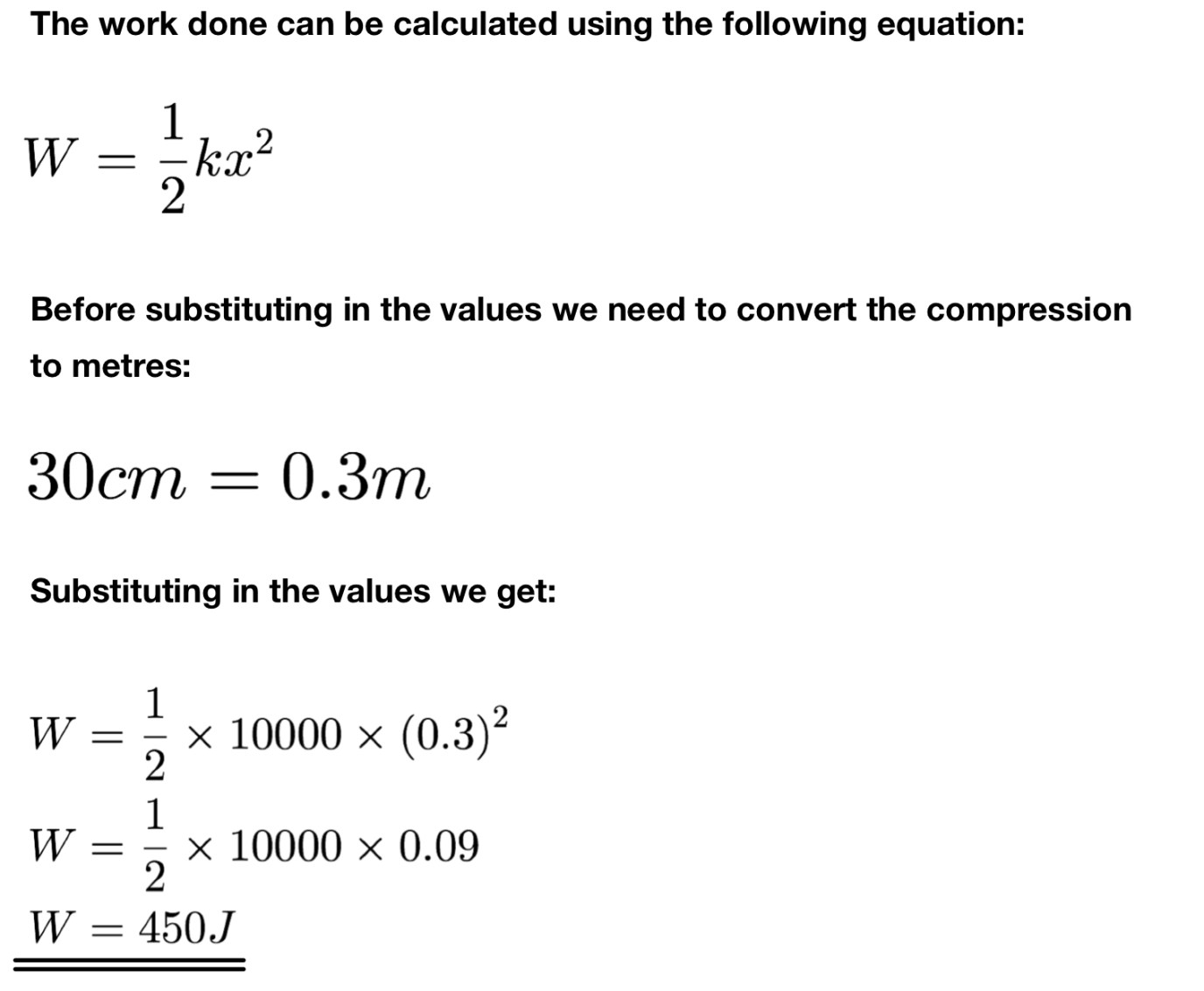
Understanding Hooke's Law is essential for anyone studying or working with materials under stress or strain. This fundamental principle in physics and engineering explains the relationship between the force applied to a spring and its resulting deformation. Central to this law is the spring constant (k), a value that determines how stiff or compliant a material is. Whether you're a student, engineer, or simply curious about how materials behave under stress, grasping the role of the k constant is key to mastering Hooke's Law. (Hooke's Law, spring constant, material deformation)
What is Hooke’s Law?

Hooke’s Law states that the force (F) required to extend or compress a spring is directly proportional to the distance (x) it is stretched or compressed from its equilibrium position. Mathematically, it is expressed as:
F = -kx,
where k is the spring constant. This law applies not only to springs but also to other elastic materials like rubber bands and metals, making it a cornerstone in material science and engineering. (elastic materials, force and displacement)
The Role of the Spring Constant (k)

The spring constant (k) is a measure of a material’s stiffness. A higher k value indicates a stiffer material that requires more force to deform, while a lower k value signifies a more flexible material. This constant is unique to each material and remains the same as long as the material behaves elastically. Understanding k is crucial for designing structures, predicting material behavior, and ensuring safety in engineering applications. (material stiffness, elastic behavior)
How to Calculate the Spring Constant (k)

To calculate the spring constant (k), you need to measure the force applied to the material and the resulting displacement. The formula is derived directly from Hooke’s Law:
k = F / x.
For example, if a force of 10 N stretches a spring by 0.5 meters, the spring constant is k = 10 N / 0.5 m = 20 N/m. This calculation is vital for material testing and engineering design. (force measurement, displacement calculation)
| Parameter | Symbol | Unit |
|---|---|---|
| Force | F | Newton (N) |
| Displacement | x | Meter (m) |
| Spring Constant | k | N/m |

📌 Note: Hooke's Law only applies within the elastic limit of a material. Beyond this limit, the material may deform permanently or break.
Applications of Hooke’s Law and the k Constant

Hooke’s Law and the k constant have numerous real-world applications, including:
- Designing suspension systems in vehicles.
- Developing medical devices like catheters and stents.
- Constructing buildings and bridges to withstand stress.
- Creating safety mechanisms like airbags and shock absorbers.
These applications highlight the importance of understanding Hooke’s Law in both theoretical and practical contexts. (engineering applications, material design)
Summary and Checklist

To summarize, Hooke’s Law is a fundamental principle that relates force and displacement in elastic materials, with the spring constant (k) playing a central role. Here’s a quick checklist to reinforce your understanding:
- Understand the formula: F = -kx.
- Calculate k using k = F / x.
- Recognize the significance of k in material stiffness.
- Apply Hooke’s Law in real-world engineering scenarios.
By mastering these concepts, you’ll be better equipped to analyze and design systems involving elastic materials. (formula application, engineering scenarios)
In conclusion, Hooke's Law and the spring constant (k) are indispensable concepts in physics and engineering. Whether you're calculating material properties or designing complex systems, understanding these principles ensures accuracy and safety. Keep exploring and applying these concepts to deepen your knowledge and expertise. (physics principles, engineering safety)
What is Hooke’s Law?
+
Hooke’s Law states that the force required to extend or compress a spring is directly proportional to the distance it is stretched or compressed, expressed as F = -kx.
How is the spring constant (k) calculated?
+
The spring constant (k) is calculated using the formula k = F / x, where F is the force applied and x is the displacement.
What does the spring constant (k) represent?
+
The spring constant (k) represents the stiffness of a material. A higher k value indicates a stiffer material, while a lower value indicates flexibility.



