Essential Math Properties Every Student Should Know

Opening Paragraph
Mathematics is the backbone of many academic and real-world applications, and understanding its foundational properties is crucial for every student. Whether you’re solving equations, analyzing data, or preparing for exams, mastering essential math properties can simplify complex problems and boost your confidence. In this blog, we’ll explore key properties like the distributive property, commutative property, and associative property, breaking them down into easy-to-understand concepts. By the end, you’ll have a clear checklist to ensure you’re equipped with the mathematical tools you need. (math properties, essential math skills, student resources)
Understanding the Commutative Property
The commutative property is one of the most fundamental math properties every student should know. It applies to both addition and multiplication, stating that changing the order of numbers does not change the result. For example:
- Addition: a + b = b + a
- Multiplication: a × b = b × a
This property is particularly useful in simplifying calculations and rearranging equations. For instance, when solving 3 + 5, you can think of it as 5 + 3 without altering the outcome.
Understanding the Associative Property
The associative property focuses on grouping numbers differently without changing the result. It applies to addition and multiplication as well:
- Addition: (a + b) + c = a + (b + c)
- Multiplication: (a × b) × c = a × (b × c)
This property is handy when dealing with multi-step problems, allowing you to group numbers in the most convenient way.
| Property | Operation | Example |
|---|---|---|
| Commutative | Addition | 4 + 5 = 5 + 4 |
| Commutative | Multiplication | 3 × 7 = 7 × 3 |
| Associative | Addition | (2 + 3) + 4 = 2 + (3 + 4) |
| Associative | Multiplication | (2 × 3) × 4 = 2 × (3 × 4) |
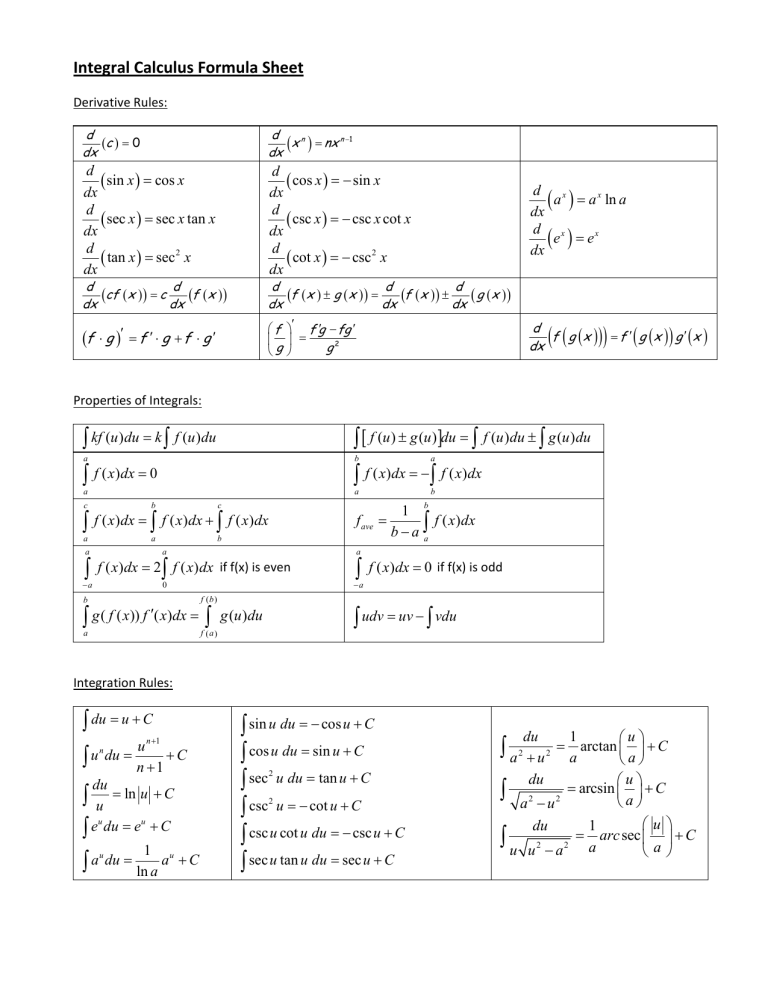
The Distributive Property Explained
The distributive property bridges the gap between addition and multiplication, allowing you to simplify expressions like a × (b + c). It’s expressed as:
- a × (b + c) = a × b + a × c
This property is essential in algebra, helping you expand and factorize equations efficiently. For example, 5 × (4 + 2) can be rewritten as 5 × 4 + 5 × 2, making it easier to solve.
💡 Note: The distributive property only works with multiplication over addition or subtraction, not with addition or subtraction over multiplication.
Identity and Inverse Properties
Two more critical properties are the identity property and the inverse property:
- Identity Property: Adding 0 or multiplying by 1 leaves a number unchanged.
- a + 0 = a
- a × 1 = a
- Inverse Property: Every number has an additive inverse (subtracting itself equals 0) and a multiplicative inverse (multiplying by its reciprocal equals 1).
- a + (-a) = 0
- a × (1/a) = 1
These properties are fundamental in solving equations and understanding number behavior.
Essential Math Properties Checklist
To ensure you’ve mastered these properties, use this checklist:
- [ ] Understand and apply the commutative property for addition and multiplication.
- [ ] Use the associative property to regroup numbers in calculations.
- [ ] Apply the distributive property to simplify expressions.
- [ ] Recognize the identity property for addition (0) and multiplication (1).
- [ ] Utilize the inverse property to solve equations.
Final Thoughts
Mastering these essential math properties is a game-changer for students at any level. They not only simplify calculations but also lay the groundwork for advanced mathematical concepts. By understanding and applying these properties, you’ll tackle problems with greater ease and confidence. Keep practicing, and soon these properties will become second nature! (math properties, essential math skills, student resources)
FAQ Section
What is the commutative property?
+
The commutative property states that changing the order of numbers in addition or multiplication does not change the result. For example, a + b = b + a and a × b = b × a.
How does the distributive property work?
+
The distributive property allows you to expand expressions by distributing multiplication over addition or subtraction. For example, a × (b + c) = a × b + a × c.
Why are math properties important for students?
+
Math properties simplify problem-solving, make calculations more efficient, and provide a foundation for advanced mathematical concepts, helping students build confidence in their skills.



