Understanding the Natural Logarithm of x Squared: ln x^2 Explained

Understanding the natural logarithm of x squared, or ln x^2, is a fundamental concept in mathematics that bridges algebra and calculus. Whether you’re a student, a professional, or simply curious about logarithmic functions, this guide will break down ln x^2 into digestible parts. By the end, you’ll grasp its definition, properties, and practical applications, empowering you to tackle related problems with confidence.
What is the Natural Logarithm of x Squared?

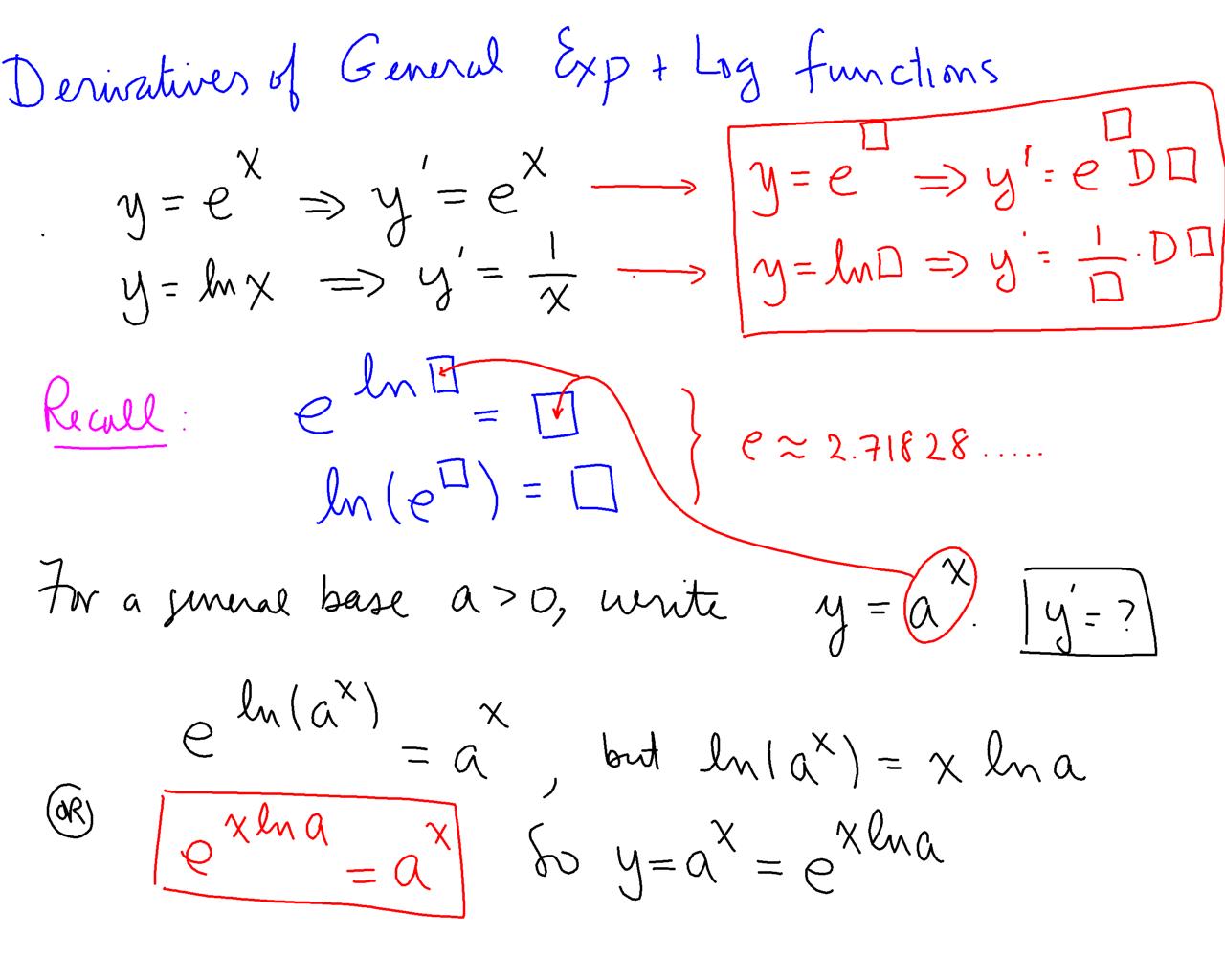
The natural logarithm, denoted as ln, is the logarithm with base e, where e is Euler’s number (approximately 2.71828). When we write ln x^2, it represents the natural logarithm of the square of x. Mathematically, it can be expressed as:
ln x^2 = 2 ln|x|
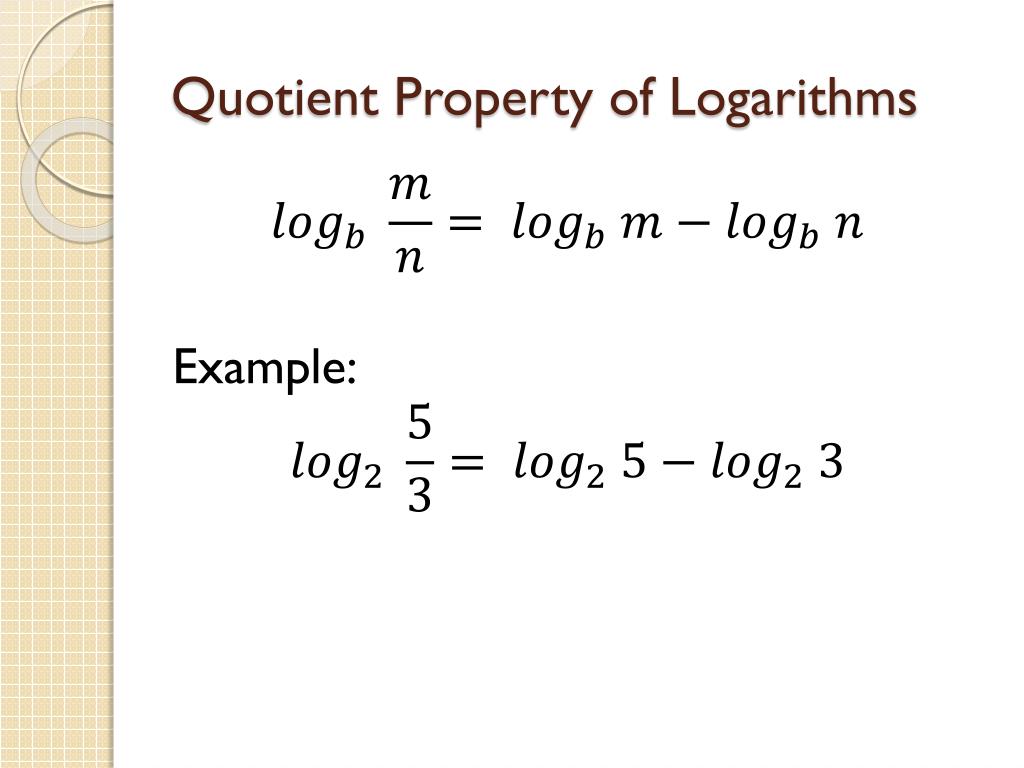
This simplification leverages the logarithmic property that states ln(a^b) = b ln(a). Here, a = x and b = 2, leading to 2 ln|x|. The absolute value ensures the expression is defined for all x ≠ 0.
📌 Note: The natural logarithm is only defined for positive real numbers, hence the absolute value in ln|x|.
Key Properties of ln x^2

Understanding ln x^2 requires familiarity with its properties. Below are the most important ones:
- Logarithmic Power Rule: ln(x^2) = 2 ln|x|.
- Domain: x > 0 or x < 0 (excluding x = 0).
- Range: All real numbers, (-∞, ∞).
- Derivative: The derivative of ln x^2 is 2/x, using the chain rule.
These properties are crucial for solving equations, integrating functions, and analyzing growth rates in real-world scenarios.
Practical Applications of ln x^2
The natural logarithm of x squared isn’t just a theoretical concept; it has practical applications in various fields:
- Physics: Modeling exponential decay or growth, such as radioactive decay.
- Economics: Analyzing compound interest or population growth.
- Engineering: Solving differential equations involving exponential functions.
| Field | Application |
|---|---|
| Physics | Radioactive decay modeling |
| Economics | Compound interest calculations |
| Engineering | Solving differential equations |
How to Solve Problems Involving ln x^2

Solving problems with ln x^2 involves applying its properties and algebraic manipulation. Here’s a step-by-step approach:
- Identify the Problem: Determine if ln x^2 is part of an equation, derivative, or integral.
- Apply Properties: Use ln(x^2) = 2 ln|x| to simplify expressions.
- Solve Algebraically: Isolate variables or solve for x as needed.
- Check Domain: Ensure x ≠ 0 to avoid undefined results.
✨ Note: Always verify your solution by substituting it back into the original equation.
Checklist for Mastering ln x^2
- Understand the definition: ln x^2 = 2 ln|x|.
- Memorize key properties: logarithmic power rule, domain, and range.
- Practice solving equations and derivatives involving ln x^2.
- Explore real-world applications to solidify your understanding.
In summary, ln x^2 is a powerful mathematical tool with wide-ranging applications. By mastering its properties and solving techniques, you’ll enhance your problem-solving skills and deepen your appreciation for logarithmic functions.
What is the natural logarithm of x squared?
+The natural logarithm of x squared, ln x^2, is equal to 2 ln|x|, using the logarithmic power rule.
Why is the absolute value used in ln|x|?
+The absolute value ensures the expression is defined for all x ≠ 0, as the natural logarithm is only defined for positive real numbers.
What is the derivative of ln x^2?
+The derivative of ln x^2 is 2/x, applying the chain rule.
natural logarithm properties, logarithmic functions, calculus tutorials, mathematical problem-solving, exponential growth models, derivative calculations, integral applications, mathematical concepts, algebra tutorials.



